报告题目:Graded geometry and applications in physics
报 告 人:Vladimir Salnikov
所在单位:CNRS/La Rochelle University
报告时间:2023年11月30日-12日1日
报告地点:313 Zhengxin Building
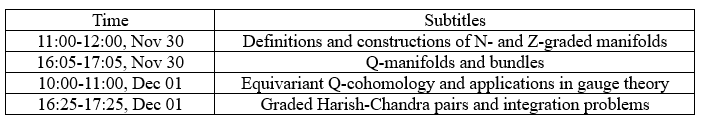
报告摘要: In this minicourse I will give an overview of results on various instances of graded geometry, that we have obtained with several colleagues in the last years. I will start with a comparison of definitions of N- and Z- graded manifolds and introduce a way of constructing a filtration of the sheaf of function on the latter one. Then I will turn to differential graded manifolds (also called Q-manifolds) and present a normal for result for a homological vector field on Z-graded manifold. Related constructions have been applied in theoretical physics, namely for studying symmetries and gauging of sigma models - I will comment on that. And in the end I will present one of my motivations to going through the labor of all these construction: the problem of integration of differential graded Lie algebras and some ongoing projects inspired by it.
报告人简介:Vladimir Salnikov is a researcher at CNRS (National Centre for Scientific Research), La Rochelle University, France. His scientific interests are graded and generalized geometry, dynamical systems, applications to mechanics and theoretical physics.